Disclaimer: These notes originates from my personal study of Schaum's Outline of Theory and Problems of College Physics 9th edition, by Frederick J. Bueche and Eugene Hecht. Images are also credited to the authors otherwise stated.
.png "physics notes | Introduction to vectors II")
From our previous lesson Physics Notes | Introduction to Vectors I we talked about scalar and vector quantities, polygon and parallelogram method of adding vectors, and subtraction of vectors. In this lesson, we focus on trigonometric ratios for solving vectors, components of vectors and unit of vectors.
Trigonometric Functions
The
trigonometric functions are defined in relation to a right angle. For the
triangle shown in figure 3, figure 1, shows the definition:
 |
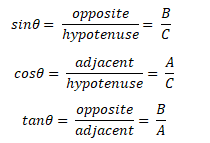| figure 1: vectors | trigonometric functions |
 |
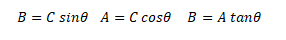| figure 2: vectors | trigonometric functions |
 |
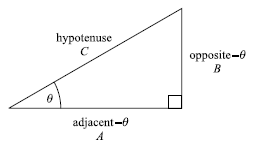| figure 3: right-angle triangle |
The trigonometric
functions are important for finding the direction of vectors. These formulas
are used when finding the direction of the resultant vector, and the components
of the resultant vector.
Component of a Vector
A
component of a vector is the useful value of a vector in a specific direction.
If an object starting from origin O
moves to a point A, as shown in the
image below, displacement has occurred and the line from O to A is called the
line of displacement (a vector). This line of displacement is parallel to the
x-axis and the y-axis, hence the x-axis is the x-component of the displacement
and the y-axis is the y-component of the displacement and the line of
displacement is known as a vector.
 |
| figure 4: components of vectors |
A vector
can be in three dimensions and two dimensions. A vector in two dimensions (as
shown above) can be resolved into two components vectors acting along any two
mutually perpendicular directions. The vector R has the x-component and y-component which are acting in mutually
perpendicular directions (acting at right angle). Hence the vector R may be resolved into the two
components Rx and Ry. The vector components Rx and Ry has magnitudes which can be found using the following formulas:
 |
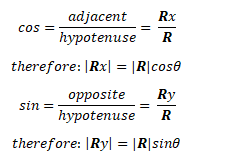| figure 5: components of vectors |
 |
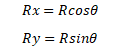| figure 6: components of vectors |
Note: The magnitude is also the size
Component Method for Adding Vectors
For a
vector with different components, the vector is resolved into each component.
If a vector has three components (x, y, and z) the vector is resolved into its
different x-component, y-component and z-component, with the positively
directed components taken as positive and the negatively directed components
taken as negative. The magnitude of x-component Rx of the resultant R is
the algebraic sum of the magnitudes of all x-components. So also the magnitude
of the y-component Ry and
z-component Rz of the resultant is
found. With the magnitude of the components known, the magnitude of the
resultant vector is given by:
 |
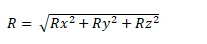| figure 7: component method for adding vectors |
With a
vector in two dimensions, the angle of the resultant R with the x-axis is given by:
 |
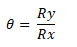| figure 8: component method for adding vectors |
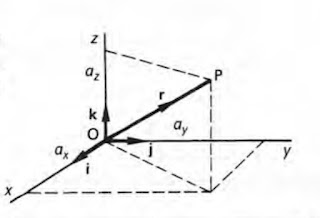
Unit Vectors
Unit vectors usually have a one magnitude and are represented by a letter symbol with a caret at the top when hand written or in text, or just a bold letter in text. The special unit vectors are i, j and k and are assigned to the x-, y- and z- axes respectively; that is, for a vector P with magnitude of x-components Rx, y-components Ry and z-components Rz; the resultant vector R can be found using the unit vectors, given by:
 |
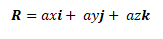| figure 9: formula for unit vectors |
The
unit vector is well illustrated with the diagram below
 |
| Image from Advance Engineering Mathematics by Stroud Ken |
A vector 3i represent a 3 unit vector in the positive x-direction and a vector of -5k represent 5 unit vector in the negative z-direction.
Displacement
Displacement
of a vector occurs when an object moves from one point (start point) to another
(end point) in space. Hence the displacement vector has an initial point (start
point) and a finial point (end point). The displacement of a vector does not
depend on the distance travelled but by the fact that the object has been
moved.
Conclusion
We have come to the end of this lesson, hope you enjoyed reading the notes:
In our next lesson, we will solve some simple and complex question on vectors which you might come across during test or exams.
Follow us on our social media handles to asked questions and also drop your suggestions on what physics topic you want to us to cover.
.png&description=Physics Notes | Introduction to Vectors II){kind=link}