Disclaimer: These notes originates from my personal study of Schaum's Outline of Theory and Problems of College Physics 9th edition, by Frederick J. Bueche and Eugene Hecht. Images are also credited to the authors otherwise stated.
 |
| physics | introduction to vectors |
Content
Scalar Quantity
A scalar
quantity is a quantity that has magnitude but not direction. Any quantity by
which the size is considered (which are usually numbers) and there is no
direction associated (such east, west, north and south), are known as scalars.
An example of a scalar quantity is the temperature. The temperature tells the
hotness or coldness of a body hence the values you can obtain is how hot or
cold a body is and the temperature will never tell you what direction (based on
the coordinate system) the hotness or coldness is going. Other examples of
scalar quantities are: length, time, temperature, mass, density, charge, speed,
distance, weight and volume. Scalar quantities are made up of ordinary numbers
by which we can perform addition and subtraction with. For example; the total
mass of the entire student in the class can be gotten by adding the mass of
each student; the total length of 5 books can be gotten by adding the length of
each book.
Vector Quantity
A vector on the other hand has magnitude and direction. That means a vector quantity has a size (which is the magnitude) and can move from one point to another (which is the direction) using the coordinate system. Examples of vector quantities are displacement, velocity, acceleration, force and momentum. I would like to say that a vector quantity combines at least two scalar quantities together or a scalar and a vector quantity. Take for example: acceleration combine distance measured in meter (m) with time given in seconds (s) hence acceleration is given by m/s² (recall that both distance and time are scalar quantity). Another example is the force which is given by the mass (m) of an object which is measured in grams and the acceleration (a) of the object which is measured in m/s². (Note that mass is a scalar quantity and acceleration is a vector quantity). An arrow drawn to scale can be used to represent a vector quantity where the length of the arrow represent the vector quantity’s magnitude, the direction the arrow head is facing represent the vector quantity’s direction.
The vector quantity is represented by a bold upper-case alphabet in text-books and when written by hand, it is represented by a small arrow drawn on the upper-case alphabet or an approximate sign under the upper-case alphabets. Symbols used to represent a vector quantity are shown below:
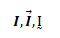 |
| symbols for vectors |
Resultant Vector
A
resultant vector is the sum of a particular type of vector. An example is the
adding together of different vector forces. The resultant force which is one
single force will have the same effect as different forces when added together.
That is, if we have different vector forces such as A, B, C, and D, the resultant force R will
be R = A+B+C+D; hence the effect of
forces A, B, C and D when combined together will be the
same effect of one single force R.
Graphical Addition of Vectors
Polygon Method for Adding Multiple Vectors
In the polygon method, the vectors to be added to find the resultant vector (R) are represented with arrows, which are drawn to scale and in the proper direction from point-to-point. That is; the end-point of one vector arrow is the beginning of another vector arrow (the tail end of each vector arrow is positioned at the tip end of the preceding one) as show below. Hence the vector arrows must be added in any order of succession such that A + B + C = C + B + A = R. The resultant vector is represented with an arrow with its tail end at the starting point and its tip end at the tip of the last vector added hence the shape formed with the added vector arrows and the resultant vector arrow is a polygon (recall that a polygon is plane shape having three or more sides). If R is the resultant vector the magnitude or size of R is given by | R |.
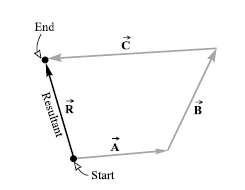 |
| Polygon Method of Vectors |
Parallelogram Method for Adding two Vectors
The resultant vector of two vectors acting at an angle may be represented by the diagonal of a parallelogram. The two vectors are drawn from the same point (origin) each facing a different direction forming a parallelogram; hence the two vectors are drawn as the sides of a parallelogram and the diagonal is the resultant vector. The direction of the resultant vector is drawn away from the origin of the two vectors. The figure below shows the parallelogram method of addition. Hence vector A and vector B are two vectors and to add both vectors together, the resultant vector R is drawn in-between the vectors, starting at the origin and ending at the point where the arbitrary lines of the vectors meet.
 |
| Parallelogram method of Vectors |
Subtraction of Vectors
Conclusion
We have come to the end of this Lesson, hope you enjoyed reading theses notes.
The next lesson will focus on different ways and formulas to solve vectors. Physics Notes | Introduction to Vectors II
In future lessons, we shall solve some vector problems you might encounter during test and exams.
Follow us on our social media handles to asked questions and also drop your suggestions on what physics topic you want to us to cover.



.png)
.png)
.png)